Back

The Hofstadter model [17–18] describes a charged particle hopping on a lattice in the presence of a magnetic field, and is noted for its fractal energy spectrum.  Recently, the model has generated renewed interest as a system that bridges the gap between the continuum quantum Hall effects and lattice-based Chern insulators. In Ref. [6] we pursue this connection in detail at the single- and many-body level.  In the process, we draw connections to recent experimental realisations of the Hofstadter model [19–20] and, significantly, make analytical progress in a field that has mostly been the subject of numerical studies. The method we present is a general approach that can be applied to a variety of interactions, lattices and parameter ranges.

Using a semiclassical analysis, we derive non-perturbative expressions for the wavefunctions, energy dispersion and Berry curvature of the low-lying bands.  As our small parameter, we use the deviation in magnetic flux density per plaquette from a simple, rational fraction (measured in units of the flux quantum).  This allows us to consider a lattice with a low flux density, which connects to the continuum limit, but also allows us to consider higher flux densities for which there is no nearby 'continuum'. In the low-density, perturbative limit we find that the lattice states and energies map smoothly onto the Landau levels of the continuum.  At higher flux densities we find a sublattice structure that maps onto several copies of a continuum Landau level. In this way, we are able to quantify the importance of lattice effects in experimental settings, as well as predict what would be observed at higher field densities.

To study the many-body physics of the model, we turn to the toolkit of Haldane pseudopotentials [21]. The many-body wavefunctions at low-field values take the form of perturbed Laughlin states that share the C₄ symmetry of the lattice. At higher magnetic field values, the ground state bosonic wavefunctions in the presence of a contact interaction become perturbed, multi-component Halperin states [22].

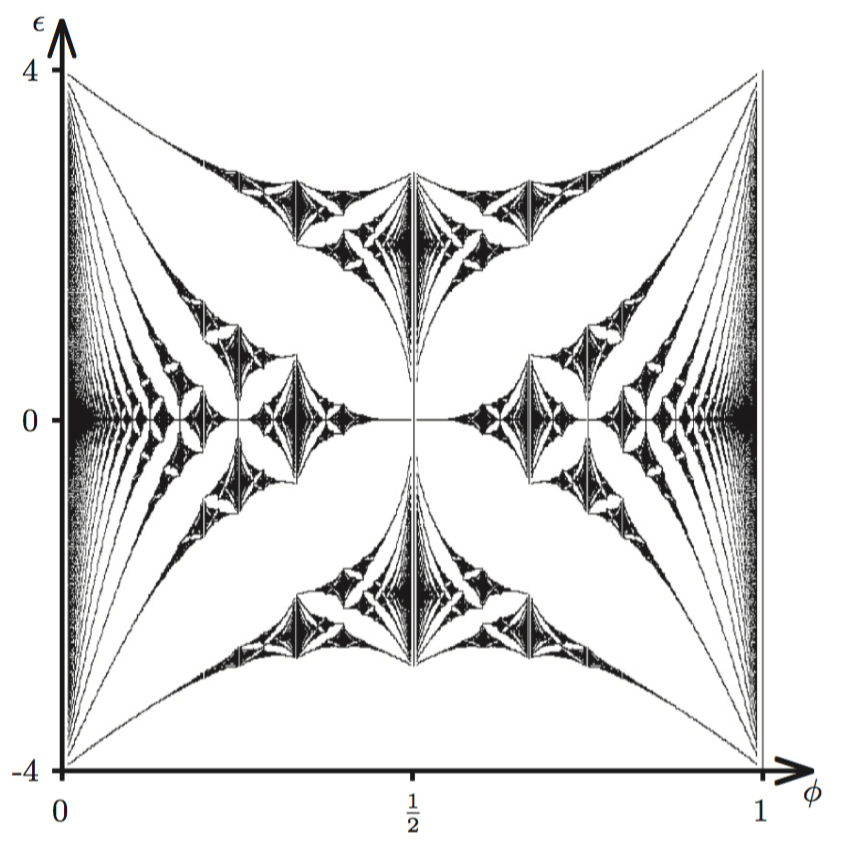
Figure 3: Hofstadter’s butterfly shows the fractal spectrum of the Hofstadter model (with energy ε) as a function of magnetic flux per plaquette, φ. The entire pattern repeats for flux filling outside of the range 0 to 1. Landau level-like lines can be observed near to the points (0, -4) and $(1/2, -2\sqrt{2})$, for example.

References

[6]     F. Harper, S. H. Simon, and R. Roy, Phys. Rev. B 90, 075104 (2014).
[17]     D. Hofstadter, Phys. Rev. B 14, 2239 (1976).
[18]    P. G. Harper, P. R. Soc. A 68, 879 (1955).
[19]    H. Miyake, G. A. Siviloglou, C. J. Kennedy, W. C. Burton, and W. Ketterle, Phys. Rev. Lett. 111, 185302 (2013).
[20]    M. Aidelsburger, M. Atala, M. Lohse, J. T. Barreiro, B. Paredes, and I. Bloch, Phys. Rev. Lett. 111, 185301 (2013).
[21]     F. D. M. Haldane, Phys. Rev. Lett. 51, 605 (1983).
[22]    B. I. Halperin, Helv. Phys. Acta 56, 75 (1983).
[23]    F. D. M. Haldane, Phys. Rev. Lett. 61, 2015 (1988).
[24]    X. Hu, M. Kargarian, and G. A. Fiete, Phys. Rev. B 84, 155116 (2011).