Back

A central principle that arises in the study of topological insulators is holography: the idea that the properties of a bulk topological phase are manifested on the boundaries of the system. This bulk-edge correspondence is neatly summarised in the celebrated periodic table of topological insulators and superconductors (TIs) [11], which classifies the bulk invariants (and associated edge modes) that may exist in a topological phase of free fermions. Notably, the edge modes of a TI in d dimensions are anomalous: the Hamiltonian that describes the physics at the boundary cannot be generated in a d-1 dimensional system. In [8] we investigated similar holographic principles in driven systems of free fermions, uncovering several new driven topological phases and unifying previous work in this field.

In a periodically driven system, the time evolution is described by a unitary operator, which derives from the underlying time-dependent Hamiltonian. In such systems, the energy of a state is no longer well defined, and states are instead labelled by a complex phase known as the quasienergy. Eigenstates, and their associated properties, may only be defined stroboscopically. 

Studies of time-dependent free-fermion systems have demonstrated the existence of edge modes that are qualitatively different from the edge modes in static TIs, such as Majorana and chiral modes at quasienergy Pi [12-15]. In these cases, the dynamical edge modes of a d-dimensional system correspond to the anomalous action of the unitary operator at the boundary, which could not arise from evolving a d-1 dimensional system.

Motivated by discussions with Ben Fregoso and Shivaji Sondhi, my coworkers and I generalized this bulk-boundary correspondence to all unitary evolutions in any dimension [8], uncovering several new dynamical topological phases in the process. We used a variant of the K-theory methods originally used by Kitaev to classify static TIs [11]. This marked a novel use of K-theory methods, which had previously only been used to classify Hamiltonians, rather than unitaries. Inspired by discussions with Graeme Segal, we mapped sets of unitaries onto sets of Hermitian maps that involved new symmetries and a new time variable. Then, with a suitable definition of stable homotopy, we constructed additive categories for each symmetry class from these Hermitian maps. This enabled us to define a K-theory in terms of quasi-surjective Banach functors between pairs of additive categories [16-17]. Finally, using the Morita equivalence of these categories, we showed that the classification of unitaries reduces to a form similar to the classification of static TIs.

We found that unitary evolutions have a twofold classification, as shown in the table below. Note that this table contains within it the periodic table of static TIs, which simply correspond to unitary evolutions with a constant Hamiltonian.

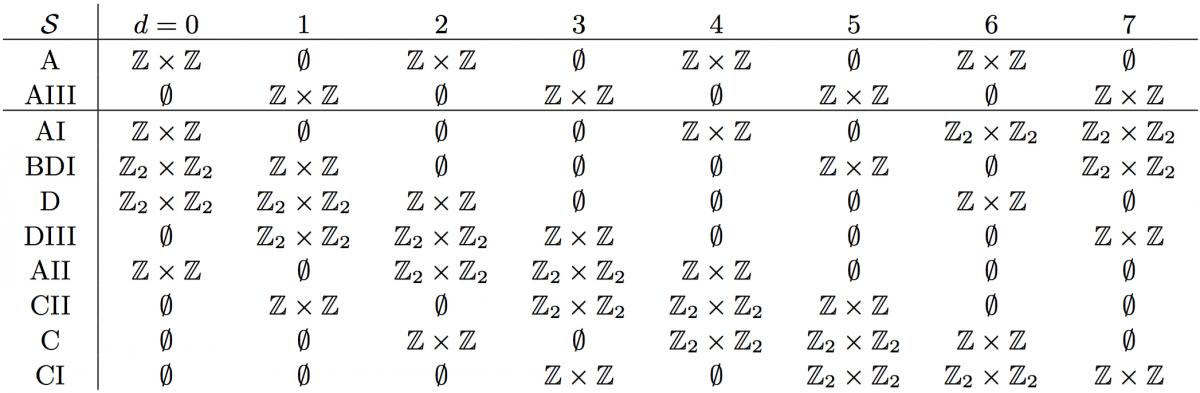
Figure 1: Classification of two-gapped unitaries by symmetry class and spatial dimension d. The table repeats for d ≥ 8 (Bott periodicity).

References

[8]     R. Roy and F. Harper, arXiv:1603.06944 (2016).
[11]     A. Kitaev, in AIP Conference Proceedings (2009) pp. 22–30.
[12]     T. Kitagawa, E. Berg, M. Rudner, and E. Demler, Phys. Rev. B 82, 235114 (2010).
[13]     L. Jiang et al., Phys. Rev. Lett. 106, 220402 (2011).
[14]     M. S. Rudner, N. H. Lindner, E. Berg, and M. Levin, Phys. Rev. X 3, 031005 (2013).
[15]     M. Thakurathi, A. A. Patel, D. Sen, and A. Dutta, Phys. Rev. B 88, 155133 (2013).
[16]     M. F. Atiyah, K-Theory (Benjamin, New York, NY, 1967).
[17]     M. Karoubi, K-Theory: An Introduction, Classics in mathematics (Springer, Berlin, 1978).